Geometric mean theorem

In Euclidean geometry, the right triangle altitude theorem or geometric mean theorem is a relation between the altitude on the hypotenuse in a right triangle and the two line segments it creates on the hypotenuse. It states that the geometric mean of the two segments equals the altitude.

Theorem and applications

If h denotes the altitude in a right triangle and p and q the segments on the hypotenuse then the theorem can be stated as:^[1]

${\displaystyle h={\sqrt {pq}}}$

or in term of areas:

${\displaystyle h^{2}=pq.}$

The latter version yields a method to square a rectangle with

Another application of provides a geometrical proof of the AM–GM inequality in the case of two numbers. For the numbers p and q one constructs a half circle with diameter p + q. Now the altitude represents the geometric mean and the radius the arithmetic mean of the two numbers. Since the altitude is always smaller or equal to the radius, this yields the inequality.^[2]

The theorem can also be thought of as a special case of the

Proof of theorem:

The triangles △ADC , △ BCD are

• consider triangles △ABC, △ACD ; here we have
  $${\displaystyle \angle ACB=\angle ADC=90^{\circ },\quad \angle BAC=\angle CAD;}$$

  
  therefore by the AA postulate
  $${\displaystyle \triangle ABC\sim \triangle ACD.}$$

  
• further, consider triangles △ABC, △BCD ; here we have
  $${\displaystyle \angle ACB=\angle BDC=90^{\circ },\quad \angle ABC=\angle CBD;}$$

  
  therefore by the AA postulate
  $${\displaystyle \triangle ABC\sim \triangle BCD.}$$

  

Therefore, both triangles △ACD, △BCD are similar to △ABC and themselves, i.e.

$${\displaystyle \triangle ACD\sim \triangle ABC\sim \triangle BCD.}$$

Because of the similarity we get the following equality of ratios and its algebraic rearrangement yields the theorem:^[1]

${\displaystyle {\frac {h}{p}}={\frac {q}{h}}\,\Leftrightarrow \,h^{2}=pq\,\Leftrightarrow \,h={\sqrt {pq}}\qquad (h,p,q>0)}$

Proof of converse:

For the converse we have a triangle △ABC in which ${\displaystyle h^{2}=pq}$ holds and need to show that the angle at C is a right angle. Now because of ${\displaystyle h^{2}=pq}$ we also have ${\displaystyle {\tfrac {h}{p}}={\tfrac {q}{h}}.}$ Together with ${\displaystyle \angle ADC=\angle CDB}$ the triangles △ADC, △BDC have an angle of equal size and have corresponding pairs of legs with the same ratio. This means the triangles are similar, which yields:

${\displaystyle {\begin{aligned}\angle ACB&=\angle ACD+\angle DCB\\&=\angle ACD+(90^{\circ }-\angle DBC)\\&=\angle ACD+(90^{\circ }-\angle ACD)\\&=90^{\circ }\end{aligned}}}$

Based on the Pythagorean theorem

In the setting of the geometric mean theorem there are three right triangles △ABC, △ADC and △DBC in which the Pythagorean theorem yields:

${\displaystyle {\begin{aligned}h^{2}&=a^{2}-q^{2}\\h^{2}&=b^{2}-p^{2}\\c^{2}&=a^{2}+b^{2}\end{aligned}}}$

Adding the first 2 two equations and then using the third then leads to:

${\displaystyle {\begin{aligned}2h^{2}&=a^{2}+b^{2}-p^{2}-q^{2}\\&=c^{2}-p^{2}-q^{2}\\&=(p+q)^{2}-p^{2}-q^{2}\\&=2pq\\\therefore \ h^{2}&=pq.\end{aligned}}}$

which finally yields the formula of the geometric mean theorem.^[4]

Based on dissection and rearrangement

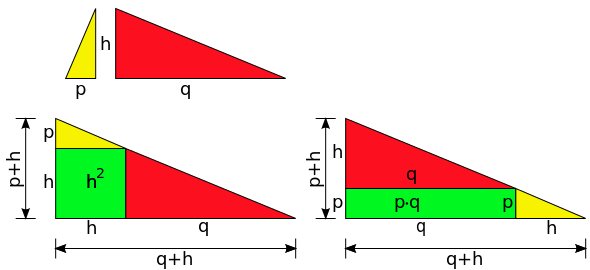
Dissecting the right triangle along its altitude h yields two similar triangles, which can be augmented and arranged in two alternative ways into a larger right triangle with perpendicular sides of lengths p + h and q + h. One such arrangement requires a square of area h² to complete it, the other a rectangle of area pq. Since both arrangements yield the same triangle, the areas of the square and the rectangle must be identical.

Based on shear mappings

The square of the altitude can be transformed into an rectangle of equal area with sides p and q with the help of three shear mappings (shear mappings preserve the area):

References

External links

• Geometric Mean at
  Cut-the-Knot

Wikimedia Commons has media related to Geometric mean theorem.