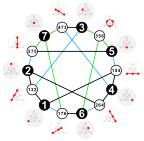
Heawood graph

Source: Wikipedia, the free encyclopedia.
Heawood graph
Hamiltonian
Symmetric
Orientably simple
Table of graphs and parameters

In the

undirected graph with 14 vertices and 21 edges, named after Percy John Heawood.[1]

Combinatorial properties

The graph is

Foster census) and therefore distance regular.[2]

There are 24

Hamiltonian cycle. For instance, the figure shows the vertices of the graph placed on a cycle, with the internal diagonals of the cycle forming a matching. By subdividing the cycle edges into two matchings, we can partition the Heawood graph into three perfect matchings (that is, 3-color its edges) in eight different ways.[2] Every two perfect matchings, and every two Hamiltonian cycles, can be transformed into each other by a symmetry of the graph.[3]

There are 28 six-vertex cycles in the Heawood graph. Each 6-cycle is disjoint from exactly three other 6-cycles; among these three 6-cycles, each one is the symmetric difference of the other two. The graph with one node per 6-cycle, and one edge for each disjoint pair of 6-cycles, is the Coxeter graph.[4]

Geometric and topological properties

Heawood's map. Opposite edges of the large hexagon are connected to form a torus.

The Heawood graph is a

coloring the map requires 7 colors. The map and graph were discovered by Percy John Heawood in 1890, who proved that no map on the torus could require more than seven colors and thus this map is maximal.[6][7]

The map can be faithfully realized as the Szilassi polyhedron,[8] the only known polyhedron apart from the tetrahedron such that every pair of faces is adjacent.

Fano plane and two representations of its Levi graph (below as a bipartite graph)

The Heawood graph is the

SL3(F2)
.

The Heawood graph has crossing number 3, and is the smallest cubic graph with that crossing number (sequence A110507 in the OEIS). Including the Heawood graph, there are 8 distinct graphs of order 14 with crossing number 3.

The Heawood graph is the smallest cubic graph with Colin de Verdière graph invariant μ = 6.[9]

The Heawood graph is a unit distance graph: it can be embedded in the plane such that adjacent vertices are exactly at distance one apart, with no two vertices embedded to the same point and no vertex embedded into a point within an edge.[10]

Algebraic properties

The automorphism group of the Heawood graph is isomorphic to the projective linear group PGL2(7), a group of order 336.[11] It acts transitively on the vertices, on the edges and on the arcs of the graph. Therefore, the Heawood graph is a symmetric graph. It has automorphisms that take any vertex to any other vertex and any edge to any other edge. More strongly, the Heawood graph is 4-arc-transitive.[12] According to the

Foster census, the Heawood graph, referenced as F014A, is the only cubic symmetric graph on 14 vertices.[13][14]

It has

book thickness 3 and queue number 2.[15]

The characteristic polynomial of the Heawood graph is . It is the only graph with this characteristic polynomial, making it a graph determined by its spectrum.

Gallery

References

  1. ^ Weisstein, Eric W. "Heawood Graph". MathWorld.
  2. ^ a b Brouwer, Andries E. "Heawood graph". Additions and Corrections to the book Distance-Regular Graphs (Brouwer, Cohen, Neumaier; Springer; 1989)
  3. MR 2099150
    .
  4. S2CID 754481
    .
  5. ^
    doi:10.1090/S0002-9904-1950-09407-5
  6. JSTOR 3219140. Archived from the original
    (PDF) on 2012-02-05. Retrieved 2006-10-27.
  7. ^ Heawood, P. J. (1890). "Map-colour theorem". Quarterly Journal of Mathematics. First Series. 24: 322–339.
  8. ^ Szilassi, Lajos (1986), "Regular toroids" (PDF), Structural Topology, 13: 69–80
  9. doi:10.1016/j.jctb.2005.09.004
    .
  10. Bibcode:2009arXiv0912.5395G
    .
  11. ISBN 0-444-19451-7. Archived from the original
    on 2010-04-13. Retrieved 2019-12-18.
  12. ^ Conder, Marston; Morton, Margaret (1995). "Classification of Trivalent Symmetric Graphs of Small Order" (PDF). Australasian Journal of Combinatorics. 11: 146.
  13. ^ Royle, G. "Cubic Symmetric Graphs (The Foster Census)." Archived 2008-07-20 at the Wayback Machine
  14. ^ Conder, M. and Dobcsányi, P. "Trivalent Symmetric Graphs Up to 768 Vertices." J. Combin. Math. Combin. Comput. 40, 41-63, 2002.
  15. ^ Jessica Wolz, Engineering Linear Layouts with SAT. Master Thesis, University of Tübingen, 2018
Wikimedia Commons has media related to Heawood graph.
Retrieved from "https://en.wikipedia.org/w/index.php?title=Heawood_graph&oldid=1205448241"