Matrix representation
Matrix representation is a method used by a
Basic mathematical operations
An m × n (read as m by n) order matrix is a set of numbers arranged in m rows and n columns. Matrices of the same order can be added by adding the corresponding elements. Two matrices can be multiplied, the condition being that the number of columns of the first matrix is equal to the number of rows of the second matrix. Hence, if an m × n matrix is multiplied with an n × r matrix, then the resultant matrix will be of the order m × r.[3]
Operations like row operations or column operations can be performed on a matrix, using which we can obtain the inverse of a matrix. The inverse may be obtained by determining the adjoint as well.[3] rows and columns are the different classes of matrices
In 3D graphics
The choice of representation for 4×4 matrices commonly used in
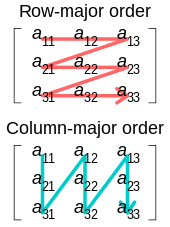
Row major (SoA)
With row-major matrix order, it is easy to transform vectors using dot product operations, since the coefficients of each component are sequential in memory. Consequently, this layout may be desirable if a processor supports dot product operations natively. It is also possible to efficiently use a '3×4' affine transformation matrix without padding or awkward permutes.
Column major (AoS)
With column-major order, a "matrix × vector" multiply can be implemented with vectorized
See also
References
- ^ "Representation of Orthogonal or Unitary Matrices". University of Texas at Austin. Retrieved 14 September 2011.
- hdl:1911/101830.
- ^ ISBN 978-0-07-063419-0.
