Harmonic analysis
Harmonic analysis is a branch of
The term "
The classical Fourier transform on Rn is still an area of ongoing research, particularly concerning Fourier transformation on more general objects such as
Fourier series can be conveniently studied in the context of Hilbert spaces, which provides a connection between harmonic analysis and functional analysis. There are four versions of the Fourier transform, dependent on the spaces that are mapped by the transformation:
- discrete/periodic–discrete/periodic: discrete Fourier transform,
- continuous/periodic–discrete/aperiodic: Fourier series,
- discrete/aperiodic–continuous/periodic: discrete-time Fourier transform,
- continuous/aperiodic–continuous/aperiodic: Fourier transform.
Abstract harmonic analysis
One of the most modern branches of harmonic analysis, having its roots in the mid-20th century, is analysis on topological groups. The core motivating ideas are the various Fourier transforms, which can be generalized to a transform of functions defined on Hausdorff locally compact topological groups.
The theory for abelian locally compact groups is called Pontryagin duality.
Harmonic analysis studies the properties of that duality. Fourier transforms and attempts to extend those features to different settings, for instance, to the case of non-abelian Lie groups.
Harmonic analysis is closely related to the theory of unitary group representations for general non-abelian locally compact groups. For compact groups, the
If the group is neither abelian nor compact, no general satisfactory theory is currently known ("satisfactory" means at least as strong as the
Other branches
- Study of the Laplacian on domains, manifolds, and (to a lesser extent) graphs is also considered a branch of harmonic analysis. See, e.g., hearing the shape of a drum.[2]
- Harmonic analysis on Euclidean spaces deals with properties of the spherical harmonics.
- Harmonic analysis on tube domains is concerned with generalizing properties of Hardy spaces to higher dimensions.
Applied harmonic analysis

Many applications of harmonic analysis in science and engineering begin with the idea or hypothesis that a phenomenon or signal is composed of a sum of individual oscillatory components. Ocean tides and vibrating strings are common and simple examples. The theoretical approach often tries to describe the system by a differential equation or system of equations to predict the essential features, including the amplitude, frequency, and phases of the oscillatory components. The specific equations depend on the field, but theories generally try to select equations that represent significant principles that are applicable.
The experimental approach is usually to acquire data that accurately quantifies the phenomenon. For example, in a study of tides, the experimentalist would acquire samples of water depth as a function of time at closely enough spaced intervals to see each oscillation and over a long enough duration that multiple oscillatory periods are likely included. In a study on vibrating strings, it is common for the experimentalist to acquire a sound waveform sampled at a rate at least twice that of the highest frequency expected and for a duration many times the period of the lowest frequency expected.
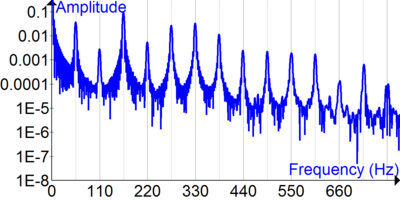
For example, the top signal at the right is a sound waveform of a bass guitar playing an open string corresponding to an A note with a fundamental frequency of 55 Hz. The waveform appears oscillatory, but it is more complex than a simple sine wave, indicating the presence of additional waves. The different wave components contributing to the sound can be revealed by applying a mathematical analysis technique known as the Fourier transform, shown in the lower figure. There is a prominent peak at 55 Hz, but other peaks at 110 Hz, 165 Hz, and at other frequencies corresponding to integer multiples of 55 Hz. In this case, 55 Hz is identified as the fundamental frequency of the string vibration, and the integer multiples are known as harmonics.
See also
- Convergence of Fourier series
- Fourier analysis for computing periodicity in evenly-spaced data
- Harmonic (mathematics)
- Least-squares spectral analysis for computing periodicity in unevenly spaced data
- Spectral density estimation
- Tate's thesis
References
- ^ "harmonic". Online Etymology Dictionary.
- ISBN 978-1461479710. Retrieved 12 December 2017.
- ^ Computed with https://sourceforge.net/projects/amoreaccuratefouriertransform/.
Bibliography
- ISBN 0-691-08078-X
- Elias Stein with Timothy S. Murphy, Harmonic Analysis: Real-Variable Methods, Orthogonality, and Oscillatory Integrals, Princeton University Press, 1993.
- Elias Stein, Topics in Harmonic Analysis Related to the Littlewood-Paley Theory, Princeton University Press, 1970.
- ISBN 0-521-83829-0; 0-521-54359-2
- Terence Tao, Fourier Transform. (Introduces the decomposition of functions into odd + even parts as a harmonic decomposition over .)
- Yurii I. Lyubich. Introduction to the Theory of Banach Representations of Groups. Translated from the 1985 Russian-language edition (Kharkov, Ukraine). Birkhäuser Verlag. 1988.
- George W. Mackey, Harmonic analysis as the exploitation of symmetry–a historical survey, Bull. Amer. Math. Soc. 3 (1980), 543–698.
- M. Bujosa, A. Bujosa and A. Garcıa-Ferrer. Mathematical Framework for Pseudo-Spectra of Linear Stochastic Difference Equations, IEEE Transactions on Signal Processing vol. 63 (2015), 6498–6509.

